Jaan Tollander de Balsch
I am a computational scientist with a background in computer science and applied mathematics. I specialize in scientific computing, installing and running software on high-performance clusters, and writing technical documentation. In the past, I have developed open-source software packages for mathematical optimization models and simulation algorithms. I am passionate about Linux, the Julia language, and command-line tools.
Mission
I believe in the growing importance of computational methods and software engineering in science and business. Given the Julia language’s combined expressive and computational power, I predict it will dominate the field of computational science in the ‘2020s. I also hope that academia will begin to focus more on developing practical software packages with modern software engineering tools instead of writing abstract white papers for the legacy publishing industry.
I view that scientific writing and open communication of scientific knowledge is essential for a functioning society. I advocate individuals to create content on platforms they own, such as personal websites, newsletters, and podcasts that can use protocols like web feeds and email for distribution instead of relying solely on third-party platforms and gated institutions.
I consider health and productivity to be the highest values of a knowledge worker. Excellent physical and mental health requires designing our environment and routines to be consistent with our evolutionary biology. For example, morning and evening walks are essential because of the circadian cycle and its effects on sleep, mood, and learning. Our tools and workspaces also need to be physically and cognitively ergonomic to reduce discomfort, which increases productivity. Productivity also requires a work environment where it is possible to focus on tasks for long periods without distractions, which is impossible in poorly designed offices.
I aim to create content that reflects my skills, values, and beliefs. My Posts focus on exploring relevant, contemporary topics related to computational science and software engineering. On the Resources page, I explore various tools, skills, and routines to become a better knowledge worker.
Posts

This article teaches you how to speed up serial algorithms by using multithreading in Julia Language. As an example, we explore how to develop a parallel version of the serial Cell Lists algorithm.
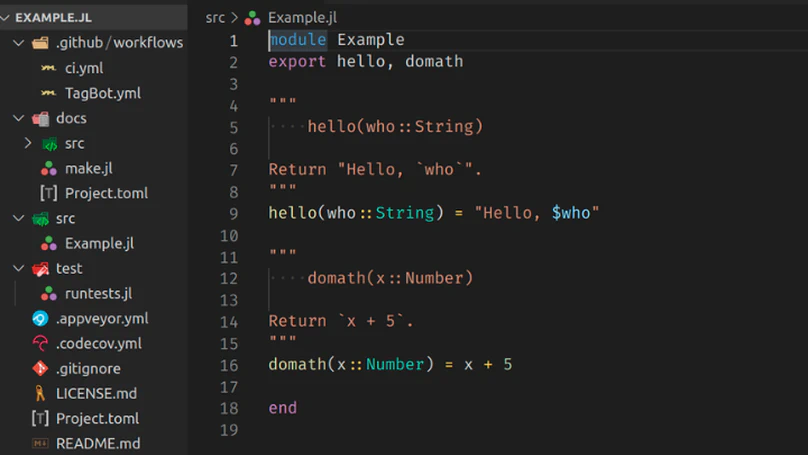
This article will teach you how to create an open-source software package in the Julia programming language and develop your package using Git-based workflows. For example, you will learn how to automate your unit tests and documentation deployment and release new versions of your package.
Videos
If you find my content useful, you may find videos on my YouTube channel helpful as well. Primarily, I create tutorial videos related to the articles and projects on my website. Also, I would appreciate it if you subscribe as a sacrificial gift for the algorithms. Thank you!
Contact
If you have questions for me, you can send me an email to the address below. Please, state your intention in the title and write your message clearly. Thank you!
